Inorganic chemistry summary
Inorganic chemistry summary
The following texts are the property of their respective authors and we thank them for giving us the opportunity to share for free to students, teachers and users of the Web their texts will used only for illustrative educational and scientific purposes only.
The information of medicine and health contained in the site are of a general nature and purpose which is purely informative and for this reason may not replace in any case, the council of a doctor or a qualified entity legally to the profession.
![]()
Inorganic chemistry summary
Chemistry
Summary inorganic chemistry part 1
 Paragraph 6.11: Coordination complexes. In a “Coordination complex” a central atom or ion is coordinated by one or more molecules or ions (ligands) which act as Lewis bases (donates electrons), forming coordinate bonds with the central atom or ion which acts as a Lewis acid (accepts electrons). Atoms in the ligands that are directly bonded are called donor atoms. A line is used to denote the interaction between an anionic (negative ion) ligand and the acceptor, an arrow is used to show the donation of an electron pair from a neutral ligand to an acceptor. The resulting species from a coordinate bond is called an adduct. Can be indicated by a dot, e.g.: H3B·THF.
Paragraph 6.11: Coordination complexes. In a “Coordination complex” a central atom or ion is coordinated by one or more molecules or ions (ligands) which act as Lewis bases (donates electrons), forming coordinate bonds with the central atom or ion which acts as a Lewis acid (accepts electrons). Atoms in the ligands that are directly bonded are called donor atoms. A line is used to denote the interaction between an anionic (negative ion) ligand and the acceptor, an arrow is used to show the donation of an electron pair from a neutral ligand to an acceptor. The resulting species from a coordinate bond is called an adduct. Can be indicated by a dot, e.g.: H3B·THF.
Neutral complexes are usually sparingly soluble in water, but readily soluble in organic solvents. The pH also has an effect, H+ can compete for the ligand, and OH- can act as ligand.
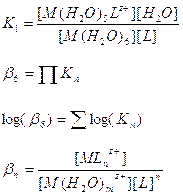 bn. Usually stability constant Kn decreases with increasing n (more ligands).
bn. Usually stability constant Kn decreases with increasing n (more ligands).
 Highly charged ions more negative ∆hydS0 because they impose more order on water, when highly charged ions form complexes charge neutralized (and also enthalpy significantly negative) → ∆G0 substantially negative.
Highly charged ions more negative ∆hydS0 because they impose more order on water, when highly charged ions form complexes charge neutralized (and also enthalpy significantly negative) → ∆G0 substantially negative.
Number of donor atoms through which ligand coordinates is called denticity of ligand (mono-, didentate etc). Polydentates → chelate ring (chelate from crab’s claw) with bite angle X-M-Y. 6 membered ring is stabilized π-bonding. Small metal ions favor 6 member rings, larger metal ions favor 5 member rings. (..) Chelate complexes more favorable than corresponding monodentate complexes (chelate effect). (..) Macrocyclic ligands: a macrocylce is a cyclic macromolecule or a macromolecular cyclic portion of a molecule.
Paragraph 19.2: Ground state e- configurations. Metals are elements that readily lose electrons to form cations. D-block metals shown in image below. A transition element (≠ d-block metal) is an atom that has incomplete d-subshell or forms cations with incomplete subshells. Each group of d-block metals consists of three members and is called a triad, first and second row metals denoted by “heavier”. First, second and third row correspond respectively filling 3d, 4d, and 5d orbitals, from which there are however minor deviations. M2+ and M3+ ions of first row metals all have [Ar]3dn.
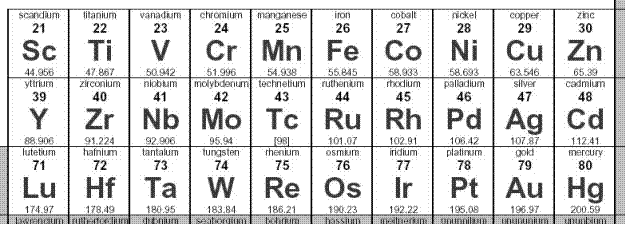
Paragraph 19.3: Physical properties. Metallic radii show little variation across a given row of d-block, the first row metal is has smallest radii, the second and third are similar. This last fact is due to the lanthanoid (La (57) – Lu (71))contraction: the steady decrease in size along the lanthanoid elements. Metals of d-block hard, ductile, malleable, less volatile then s-block. All 3d metals have values of IE1 and IE2 larger than those of calcium and all (except zinc) have have higher values of ΔaH0, which make them less reactive than calcium. Since all known M2+ ions of 3d metals are smaller than Ca2+ lattice and solvation energy effects are more favorable for 3d metal ions.
Paragraph 19.4: Reactivity. Metals are moderately reactive. On thermodynamic grounds metals should liberate H2 from acids, but generally do not either because passivation by thin surface coating of oxide or because they have a dihydrogen overpotential, or both. Silver, gold and mercury least reactive metals.
Paragraph 19.5: Characteristic properties. Colors are specific for species with other ground state than d0 and d10 and are pale because they’re against the Laporte selection rule: e- transitions only if Δl = ±1. More intenser colors originate from charge transfer absorptions or emissions. EXPAND WITH SECTION 16.4.
(..) (paramagnetism). Interconversion between oxidation states characteristic of d-block metals. Apparent oxidation state from molecular or empirical formula may be misleading, e.g. La3+(I-)2(e-), sometimes metal-metal bonds or ambiguous oxidation states, e.g. [Ti(bpy)3]n- (n = 0, 1, 2).
Paragraph 19.6: Electroneutrality. Pauling’s electroneutrality principle estimates charge distribution by assuming charge on single atom only -1 to +1. ó
Paragraph 19.7: Coordination numbers. Coordination numbers and geometries are often distorted from regular geometries, because e.g. steric effects. If there’s a small energy difference between geometries, fluxional behaviour in solution may be observed.
In the Kepert model the metal lies at the centre of a sphere over which the ligands are free to move. The ligands are considered to repel each other like VSEPR, bot non-bonding electrons are ignored, and the geometry is thus independent of the ground state e- configuration. Common arrangement in the table on the right, not all are predicted by Kepert because electronic factors or the inherent constraints of ligands. Tripodal ligands are ligands containing three arms, each with a donor atom, originating from central atom or group which also may be donor.
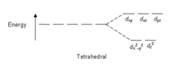
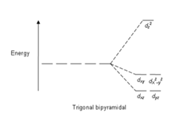
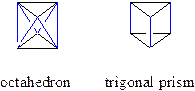 Coordination number 2. Uncommon, restricted only a few metal-ions (d10). Coorindation number 3. Also uncommon, also involving d10. Some p-block have t-shaped molecules because stereochemically active lone pairs, but not seen d-block. Coordination number 4. Are very common, mostly tetrahedral: d0,1,2,5,6,7,8,9,10. Square planar if electronic factors strongly favor square planar arrangement, usually d8. Coordination number 5. Trigonal bipyramid and square based pyramidal. Since small energy difference often between extremes. Coordination number 6. Almost always octahedral, but d4 and d9 tetragonally distorted: elongated or squashed. This is called the Jahn-Teller effect. Also a small group of d0 and d1 trigonal prismatic or distorted trigonal prismatic environment. Coordination number 7. Early second & third row (and also lanthanoids and actinoids), rcation must be relatively large. In reality much distortion from these structures. Coordination numbers 8, 9 and 10. (..)
Coordination number 2. Uncommon, restricted only a few metal-ions (d10). Coorindation number 3. Also uncommon, also involving d10. Some p-block have t-shaped molecules because stereochemically active lone pairs, but not seen d-block. Coordination number 4. Are very common, mostly tetrahedral: d0,1,2,5,6,7,8,9,10. Square planar if electronic factors strongly favor square planar arrangement, usually d8. Coordination number 5. Trigonal bipyramid and square based pyramidal. Since small energy difference often between extremes. Coordination number 6. Almost always octahedral, but d4 and d9 tetragonally distorted: elongated or squashed. This is called the Jahn-Teller effect. Also a small group of d0 and d1 trigonal prismatic or distorted trigonal prismatic environment. Coordination number 7. Early second & third row (and also lanthanoids and actinoids), rcation must be relatively large. In reality much distortion from these structures. Coordination numbers 8, 9 and 10. (..)
Paragraph 19.8: Isomerism. (..) (Read blue boxes).
Ligands |
Geometry |
Hybridization |
2 |
linear |
sp |
3 |
trigonal planar |
sp2 |
4 |
tetrahedral; |
sp3 |
4 |
square planar |
sp2d |
5 |
trigonal bipyramidal |
sp3d |
5 |
square based pyramidal |
sp3d |
6 |
octahedral |
sp3d2 |
7 |
pentagonal bipyramidal |
sp3d3 |
8 |
dodecahedral |
sp3d4 |
8 |
square antiprismatic |
sp3d4 |
8 |
hexagonal bipyrimidal |
? |
9 |
tricapped trigonal prismatic |
sp3d5 |
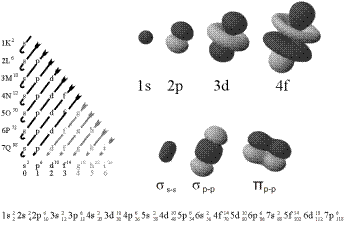
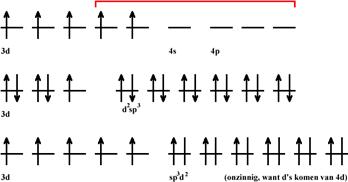
Paragraph 20.2: valence bond theory. Valence bond theory (hybridization and overlap): hybridization schemes can be used to describe bonding d-block metals. But valence bond theory is very unrealistic when trying to describe metal complexes. See third electron configuration, where electrons are put unpaired in 3d shell to achieve diamagnetism (or high spin) and 4d shell thus has to be used for hybridization.
 Paragraph 20.3: crystal field theory. Ligands are considered points charges and there are no covalent metal-ligand interactions. Electrostatic field = crystal field. See fig. 20.2, if spherical approach ligands, all 3d orbitals would be raised. But octahedral approach: dz2 and dx2-y2 raised more than dyz, dyx and dzx (the closer the ligand, the greater the raise in energy, - ↔ - repulsion). The separation energy is Δoct, because the total energy remains the same, and two and three orbitals are at the same energy level, energy is splitted 0.6 and 0.4 Δoct. Magnitude of Δoct is determined by the strength of the crystal field: Δoct(strong field) > Δoct(weak field). With absporption spectrum ion, e- promotion from t2g to eg (the resulting orbitals from splitting the d-orbital) can be seen and Δoct can thus be estimated. Δoct determined by identity and oxidation metal and nature of ligands. Δoct depends on ligands as follows:
Paragraph 20.3: crystal field theory. Ligands are considered points charges and there are no covalent metal-ligand interactions. Electrostatic field = crystal field. See fig. 20.2, if spherical approach ligands, all 3d orbitals would be raised. But octahedral approach: dz2 and dx2-y2 raised more than dyz, dyx and dzx (the closer the ligand, the greater the raise in energy, - ↔ - repulsion). The separation energy is Δoct, because the total energy remains the same, and two and three orbitals are at the same energy level, energy is splitted 0.6 and 0.4 Δoct. Magnitude of Δoct is determined by the strength of the crystal field: Δoct(strong field) > Δoct(weak field). With absporption spectrum ion, e- promotion from t2g to eg (the resulting orbitals from splitting the d-orbital) can be seen and Δoct can thus be estimated. Δoct determined by identity and oxidation metal and nature of ligands. Δoct depends on ligands as follows:
[weak field: Δoct↓] I− < Br− < S2− < SCN− < Cl− < NO3− < N3− < F− < OH− < C2O42− < H2O < NCS− < CH3CN < py (pyridine) < NH3 < en (ethylenediamine) < bipy (2,2'-bipyridine) < phen (1,10-phenanthroline) < NO2− < PPh3 < CN− < CO [strong field: Δoct↑] |
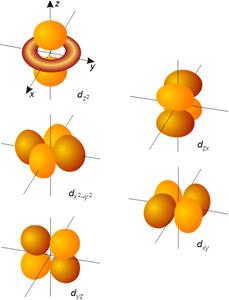 Same donor atoms close together. Δoct varies irregularly across the first row of the d-block. For metals, Δoct decreases down triad, spectrochemical series of metals can be made independent of ligands:
Same donor atoms close together. Δoct varies irregularly across the first row of the d-block. For metals, Δoct decreases down triad, spectrochemical series of metals can be made independent of ligands:
[weak field: Δoct↓] Mn(II) < Ni(II) < Co(II) < Fe(III) < Cr(III) < Co(III) < Ru(III) < Mo(III) < Rh(III) < Pd(II) < Ir(III) < Pt(IV) [strong field: Δoct↑].
Δo increases with oxidation state.
Δo decreases within group
For a given dn configuration the crystal field stabilization energy (CFSE) is the difference in energy between the d electrons in an octahedral crystal field and the d electrons if they would be located in a spherical crystal field.
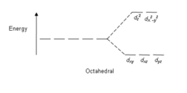
 This energy is comprised of the (loss of) exchange energy and the coulombic repulsion between the spin paired electrons. Exchange energy (see orbital image right): difference both electrons parallel spin (more stabile) and reverse spins.
This energy is comprised of the (loss of) exchange energy and the coulombic repulsion between the spin paired electrons. Exchange energy (see orbital image right): difference both electrons parallel spin (more stabile) and reverse spins.
Electrons can be put in the two splitted orbitals “low spin” or “high spin”. In low spin the lowest orbitals gets filled completely first, and in high spin the aufbau principle goes for all orbitals. The electrons will fill the orbitals creating the lowest CFSE, which is thus:
CFSE = (nlow) · Δoct,low + (nhigh) · Δoct,high – P
*where P is the # of paired in comparison with spherical field!
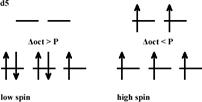 Hence, or looked upon differently, if Δoct > P (strong crystal field) it costs more energy to put the electron in the high energy orbital (eg) than paired in the lower t2g orbital, the complex will thus be low spin. The other way around: if Δoct < P (weak crystal field), it is more energy efficient to put the electrons in the higher orbital (eg) first, if these are empty.
Hence, or looked upon differently, if Δoct > P (strong crystal field) it costs more energy to put the electron in the high energy orbital (eg) than paired in the lower t2g orbital, the complex will thus be low spin. The other way around: if Δoct < P (weak crystal field), it is more energy efficient to put the electrons in the higher orbital (eg) first, if these are empty.

Jahn-Teller distortions. Jahn-Teller distortions originate if electron density is not equally distributed. The metal-ligand bond lengths are stretched if nearby orbitals are filled and vice versa (see image), leading to a distortion. This is often the case in octahedral d4 and d9 complexes.
The Jahn-Teller theorem states that any non-linear molecular system in a degenerate electronic state will be unstable and will undergo distortion to form a system of lower symmetry and lower energy, thereby removing the degeneracy.
 Tetrahedral crystal field. dxy, dyz, dxz orbitals nearer to ligands than dz2 and dx2-y2. Δtet = 4/9·Δoct, because Δtet is smaller, tetrahedral complexes are high spin. Also different colours. In FeO44- Jahn-Teller distortions lead to different bond angles.
Tetrahedral crystal field. dxy, dyz, dxz orbitals nearer to ligands than dz2 and dx2-y2. Δtet = 4/9·Δoct, because Δtet is smaller, tetrahedral complexes are high spin. Also different colours. In FeO44- Jahn-Teller distortions lead to different bond angles.
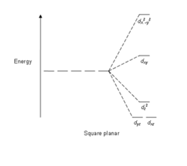
Square planar crystal field. Can be derived by removing two trans ligands from an octahedral configuration. E.g. from z-axis, dz2 greatly stabilized, dyz and dxz (also point partially in z-direction) also stabilized, dx2-y2 is massively destabilized, whereas dxy is moderately destabilized. [NiCl4]2- is tetrahedral, while [Pd(II)X4]z- and [Pt(II)X4]z- are both square planar, because Pd and Pt (2nd and 3rd row) cause larger crystal fields.
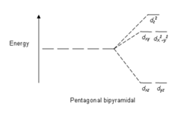
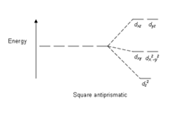
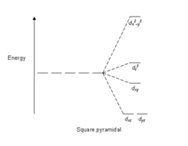
Other crystal fields. See image on next page, though only for like ligands!
Octahedral |
Pentagonal bipyramidal |
Square antiprismatic |
|
|
|
Square planar |
Square pyramidal |
Tetrahedral |
|
|
|
Trigonal bipyramidal |
|
|
|
|
|
Paragraph 20.4: Molecular orbital theory. Molecular orbital theory offers alternative for the crystal field theory. First take a look at §3.2 and pp 109.
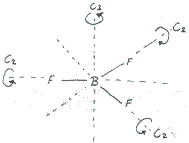
Paragraph 3.2: Symmetry operations & elements. A symmetry operation is an operation which leaves it in a configuration that is indistinguishable from its original configuration. The operation is carried out with respect to symmetry elements, i.e. points, lines or planes. The symmetry operation around an n-fold axis is noted by Cn, n-fold meaning that a (360°/n) rotation leads to the same configuration. If a molecule possesses more than one axis, the axis with the highest n-value is called the principal axis. Sometimes different order axes coincide (e.g. C4 with C2 in square planar).
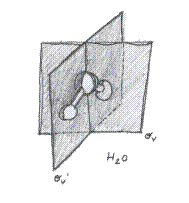
Reflection through a plane is denoted with the σ symbol. If the plane is perpendicular to a Cn axis, it is labelled σh (horizontal) and if it coincides with the principal axis it is labeled σv (vertical). σv refers to the plane bisecting the (H-O-H) bond and σv’ refers to the plane in which the molecule lies. A σd (dihedral) label is given if the plane bisects an angle between two Cn axes.
The center of inversion is the point from which you can draw an infinite number of straight lines such that each line passes through (a) pair(s) of similar points, one on each side of the centre of symmetry and at equal distance from it.
A rotation followed by a reflection through a plane perpendicular to that axes, which is called the improper rotation axis, resulting in the same configuration, is designated with Sn.
All objects can be operated upon by identity operater E, which leaves the molecule unchanged, all elements are thus at least said to have the symmetry element E.
Page 109: A bent triatomic: H2O. Symmetry can be used in different ways, a short example follows.
C2v |
E |
C2 |
σv(xz) |
σv’(yz) |
A1 |
1 |
1 |
1 |
1 |
A2 |
1 |
1 |
-1 |
-1 |
B1 |
1 |
-1 |
1 |
-1 |
B2 |
1 |
-1 |
-1 |
1 |
It is possible to develop an MO picture of the H2O bonding based upon symmetry information. The “C2v character table” is shown above: labels in the first column under the “point group symbol” tell us the symmetry types of orbitals that are permitted within the specified point group. A point group is a set of symmetry operations forming a mathematical group, for which at least one point remains fixed under all operations of the group . The numbers in the E column indicate the degeneracy of each type of orbital, in the C2v point group all orbitals have a degeneracy of 1, i.e. they are non-degenerate. The rows of numbers following a given symmetry label indicate how a particular orbital behaves when operated by each symmetry operation: 1 means unchanged, -1 means it changes sign, 0 means it changes in some other way. For example: the 2s orbital remains unchanged by all symmetry operations (resulting in only “ones”), and is as defined in the character table given the label a1 (lower case, only in the table uppercase letters are used).
Symbol |
Operation |
Element |
Example |
Cn |
Rotation |
n-fold axis (360°/n) |
|
σ |
Reflection |
Plane |
|
i |
|
Centre |
|
Sn |
Rotation + reflection through a plane perpendicular to the axis |
n-fold improper rotation axis |
|
Paragraph 20.4: Molecular orbital theory (continued). MO theory does consider covalent interactions in complexes.
MO theory (based on chapter 4 – p 120: SF6)
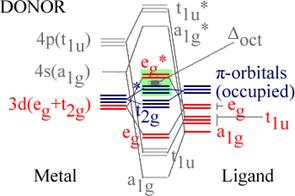
MO theory compares symmetries to establish a molecular orbital diagram. For example, a first row metal has 3d, 4s and 4p valence shells. Those can be divided in different symmetries.
It is known that only p orbitals of the ligands interact with the metal. More precise, only the pz orbitals (if each ligand is given its own axis set). Furthermore, from an examination of how many of these p orbitals are the same after Oh symmetry operations it can be concluded that the LGO is a sum of A1g, T1u and Eg symmetries. 6 new wavefunctions can thus be derived by mixing the p orbitals.
|
σ-bonding only. For an octahedral metal-ligand complex an MO diagram can thus be constructed, each ligand behaves similar to the aforementioned fluor. The metal has five 3d orbitals (eg and t2g), a 4s orbital (a1g) and three 4p orbitals (t1u). This leads to the following MO diagram:
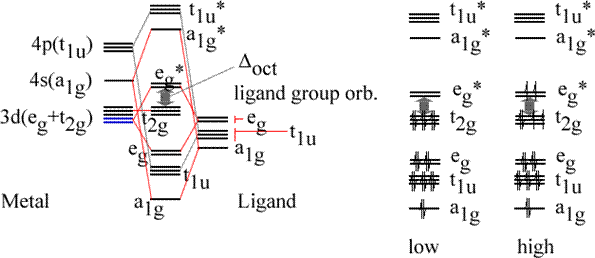
There is greater overlap between the metal s and p orbitals and the ligand p orbitals than between the metal 3d and the ligand p, which leads to more stabilization. If there is no π bonding, the energy levels between t2g and eg* correspond with Δoct. Obviously, if the eg, t1u and a1g orbitals are filled (which can be done by the electrons supplied by the ligands), the remaining electrons (from the metal) are divided depending on weak or strong field, just as in the crystal field theory.
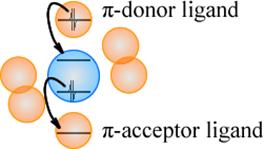 Complexes with π bonding also. Sometimes π bonding considered for d t2g orbitals (dxy, dxz, dyz) and ligand d orbitals in the case of phosphine ligands (e.g. PR3 or PF3), but more often other interactions. I.e. ligand σ*-orbitals as acceptor orbitals. There are two types of ligands: π-donor and π-acceptor ligands.
Complexes with π bonding also. Sometimes π bonding considered for d t2g orbitals (dxy, dxz, dyz) and ligand d orbitals in the case of phosphine ligands (e.g. PR3 or PF3), but more often other interactions. I.e. ligand σ*-orbitals as acceptor orbitals. There are two types of ligands: π-donor and π-acceptor ligands.
- a π-donor ligand donates electrons to metal centre in an interaction that involves a filled ligand orbital and an empty metal orbital.
- a π-acceptor ligand accepts electrons from the metal centre in an interaction that involves a filled metal orbital and an empty ligand orbital.
π-acceptor ligands can stabilize low oxidation state metal complexes.
π-donor ligands |
π-acceptor ligand |
Cl-, Br-, I- |
CO, N2, NO |
When a metal is bound to a π-donor ligand, the t2g orbitals will not be split very far. When a metal is bound to a π-acceptor ligand, the splitting will be much more. See diagrams above.
Also, when the metal is bound to a π-donor ligand, these orbitals will bring in more electrons, thus changing “where the metal electrons will go” (marked light green; the rest is filled with bonding electrons, in case of donor thus also donated electrons). The number of bonding electrons is always the same in the two separate cases respectively, the number of metal electrons can change: in σ-complexes there are 12 electrons from the ligand and in π-complexes the σ and π orbitals from the ligand contribute 18 electrons (6*2σ + 6π). These can be considered to fill the orbitals below the “green orbitals” (see picture).
For better, but still qualitative pictures, see p. 568 book. However, conclusions can be drawn:
- Δoct decreases in going from σ-only complex to σ- and π-complex (compare above donor picture to picture on previous page; and bare in mind that eg* stays equal).
- For a complex with π-donor ligands, increased π-donation (?) stabilizes t2g level and destabilizes t2g*, thus decreasing Δoct.
- Δoct values are relatively large for π-acceptor ligands, and those complexes are thus likely to be low spin.
- For a complex with π-acceptor ligands, increased π-acceptance stabilizes t2g, increasing Δoct.
Furthermore, since filling antibonding orbitals is detrimental for complex-formation, octahedral complexes with π-accepting ligands will not be favoured by dn>6. An observation can be made that d-block metals tend to obey the “effective atomic number rule” or the “18-electron rule”. See also chapter 23: A low oxidation state organometallic complex contains π-acceptor ligands and the metal centre tends to acquire 18 electrons in its valence shell (the “18-electron rule”), thus filling the valance shell. The 18-eletron rule is useless for higher oxidation state metals, can be rationalized by smaller energy seperations. Other complexes than octahedral fall out of the scope of this discussion (see p. 570).
(..; some exceptions for NO).
Paragraph 20.5: Ligand field theory. Won’t go in to mathematics of ligand field theory, however, ligand field theory is an extension to crystal field theory which is freely parameterized (as opposed to localized field from point charge). It is also confined to d orbitals. Apart from Δoct it also uses “Racah” parameters which are obtained from electronic spectroscopic data.
Paragraph 20.6: Electronic spectra. Absorptions arise from transition between electronic energy levels:
- Transitions between metal-centred orbitals possessing d-character (‘d-d’ transitions).
- Weaker.
- Can be masked due CT transition.
- Transitions between metal- and ligand-centred MOs which transfer charge from metal to ligand or vice versa.
- More intense.
- MLCT: metal-to-ligand charge transfer
- LMCT: ligand-to-metal charge transfer
 is the wavenumber.
is the wavenumber.
Absorptions are relatively fast in comparison to molecular vibrations and rotations (with which the energy levels change), hence, the observed absorption frequencies vary. There is an absorption maximum λmax (nm), with corresponding Amax (absorbance), λmax is used to describe the particular band. The molar extinction coefficient (aka molar absorptivity) εmax:
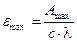 Where εmax is the molar extinction coefficient, Amax the maximum absorption (@ λmax), c the concentration, and l the pathlength (in cm!) of the spectrometer cell.
Where εmax is the molar extinction coefficient, Amax the maximum absorption (@ λmax), c the concentration, and l the pathlength (in cm!) of the spectrometer cell.
Note (without explanation) that:
- d1,4,6,9 complexes consist of one absorption.
- d2,3,7,8 copmlexes consist of three absorptions.
- d5 complexes consist of a series of weak but sharp absorptions.
Selection rules.
Spin selection rule: #paired/unpaired electrons stays equal. Spin quantum number S doesn’t change (ΔS = 0).
Laporte selection rule: Orbital quantum number l has to change with 1: Δl = 0 (e.g. s→s and p→f forbidden).
These selection rules are strict, but exceptions occur under specific conditions (see p. 571):
Type |
Typical εmax |
Spin forbidden, ‘d-d’ |
<1 |
Laporte forbidden, Spin allowed ‘d-d’ |
1-10 (centrosymmetrisch), 10 -1000 (niet centrosymmetrisch) |
Charge transfer (fully allowed) |
1000 - 50 000 |
 Paragraph 20.8: Magnetic properties. Paramagnetism arises from unpaired electrons. Each electron has a magnetic moment with one component associated with spin angular momentum of electron, and a second component associated with the orbital angular momentum (except when l = 0 (s)). The image to the right depicts momenta, however, this is not realistic, “spins are not real”.
Paragraph 20.8: Magnetic properties. Paramagnetism arises from unpaired electrons. Each electron has a magnetic moment with one component associated with spin angular momentum of electron, and a second component associated with the orbital angular momentum (except when l = 0 (s)). The image to the right depicts momenta, however, this is not realistic, “spins are not real”.
For many d-block complexes the orbital momentum can be ignored, and the magnetic moment μ can be determined from the number of unpaired electrons:
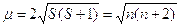
Where S = total spin quantum number and n = unpaired electrons (S = n/2).
Effective magnetic moment, μeff, can be obtained from experimentally measured molar magnetic susceptibility, χm. The μeff can be determined from χm as follows:
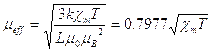 (for S.I. units).
(for S.I. units).
k = Boltzman, χm = magnetic susceptibility, T = temperature, L = Avagadro number, μ0 = vacuum permeability, μB = “Bohr magneton” (see p. 579).
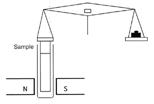
E.g. a Gouy balance: sample is hung in balance and magnetic field applied to it, sample moves and difference in weight can be read out, from which magnetic susceptibility, χm, can be determined.
Ferromagnetism, antiferromagnetism and ferrimagnetism. If metal centres are separated by diamagnetic species, they are said to be “magnetically dilute”. When paramagnetic species are close together (bulk metal), or separated by species able to transmit magnetic interactions (as many d-block oxides, fluorides and chlorides are), the metal centres can “couple” (interact). This may give rise to ferromagnetism or antiferromagnetism.
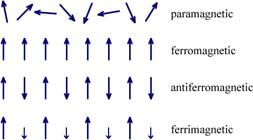 Above the Curie temperature (Tc) the thermal energy is sufficient to overcome the alignment. Antiferromagnetism occurs below the Néel temperature (TN). Ferrimagnetism occurs when relative values of momenta are different.
Above the Curie temperature (Tc) the thermal energy is sufficient to overcome the alignment. Antiferromagnetism occurs below the Néel temperature (TN). Ferrimagnetism occurs when relative values of momenta are different.
When a bridging ligand facilitates coupling of electron spins on adjacent metal centres, this happens by super-exchange. The two metal centres (in image on the left and right) interact with two spin-paired electrons in the same orbital of a ligand. Since these are spin paired, and the configuration in the MO also has to be spin-paired, the result is an anti-parallel coupling of the two metal centres.
Paragraph 20.9: ligand field stabilization energies (LFSE). LFSE terms are only small parts of total interaction energy. However, going to the different dn configurations, it shows remarkable similarities with other thermodynamic energy trends, such as lattice energies and hydration
enthalpies of metals. Deviation from the dotted line may thus be taken as measures of “thermochemical LFSE” values. Generally LFSE values are in compliance with Δoct values measured from electronic spectroscopy.
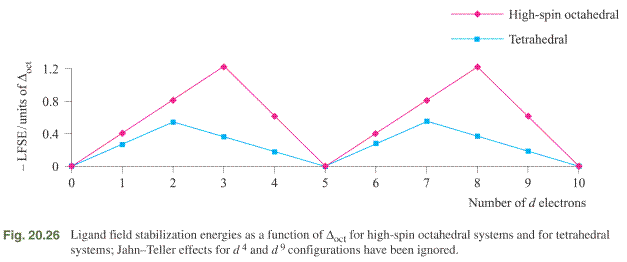
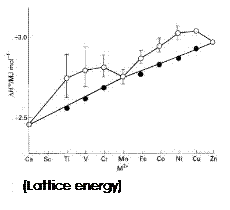
As can be seen the figure above, d0,5,10 complexes should not favour octahedral or tetrahedral configurations. However, other factors should be taken into account. E.g. the smaller size of tetrahedral complexes results in higher lattice and salvation energies, hence, for example Ni2+ (d8) does not form tetrahedral complexes in aqueous solutions, only in melts or non-aqueous media.
(..; verhaal over “spinels”).
Paragraph 20.10: The Irving-Williams series. In aqueous solutions, water is replaced by other ligands, and the position of this equilibrium will be related to the difference between the two LFSEs, since Δoct is ligand-dependent. The stability constants however show the following single-hump:
Mn(II) |
< |
Fe(II) |
< |
Co(II) |
< |
Ni(II) |
< |
Cu(II) |
> |
Zn(II) |
d5 |
|
d6 |
|
d7 |
|
d8 |
|
d9 |
|
d10 |
This is not in accordance with the LFSE energies (that peak at d8), but this can be explained from the fact that LFSE is not the only contributing factor. Ionic radii should also be taken into account, these have the following trend:
Mn(II) |
> |
Fe(II) |
> |
Co(II) |
> |
Ni(II) |
< |
Cu(II) |
< |
Zn(II) |
d5 |
|
d6 |
|
d7 |
|
d8 |
|
d9 |
|
d10 |
This trend can also be explained by dependency on dn configurations (and not only on the Zeff increment leading to a decrease in radius). However, this trend also doesn’t explain the stability of copper. Copper is very stabile because a d9 complex has a Jahn-Teller distortion. This also renders the image of a fixed ionic radius useless. Appearantly in this distortions 4 stronger bonds compensate for the 2 weaker (longer) bonds in such a way to make it more stabile than Ni(II).
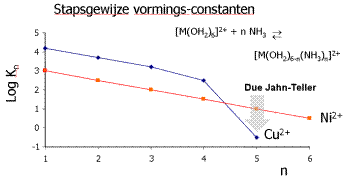
Paragraph 20.11: oxidation states in aqueous solutions. E0 values (..; nog lezen!- na “deel 2”).
Ook nog doen:
- Chelaat effect meer uitleg *zie A38
Source: http://www.jintram.nl/bestanden/SRM4/Summary%20inorganic%20chemistry%20_%20v0.05.doc
Google key word : Inorganic chemistry summary file type : doc
Author : not indicated on the source document of the above text
If you are the author of the text above and you not agree to share your knowledge for teaching, research, scholarship (for fair use as indicated in the United States copyrigh low) please send us an e-mail and we will remove your text quickly.
Inorganic chemistry summary
If you want to quickly find the pages about a particular topic as Inorganic chemistry summary use the following search engine:
Chemistry
Inorganic chemistry summary
Please visit our home page
Larapedia.com Terms of service and privacy page


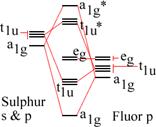 To construct the MO diagram, orbitals of same symmetry are mixed. Orbitals with another symmetry become non-bonding orbitals. Displayed to the left an image of bonding in SF6. S only has s & p orbitals.
To construct the MO diagram, orbitals of same symmetry are mixed. Orbitals with another symmetry become non-bonding orbitals. Displayed to the left an image of bonding in SF6. S only has s & p orbitals.